Explain Differences Negative Squre Root Positive

By Kur_164Abbigail 18 May, 2022 Post a Comment

The negative solution of x 2 a is a we know that if x satisfies x 2 a then x 2 x 2 a therefore because a is a solution so is. In reality all the positive numbers have two square roots a positive and a negative.

Exponents And Square Roots Gre Video Lessons Examples And Solutions

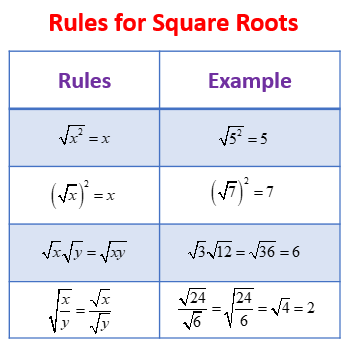
The principal square root of latexalatex is.

. For example the square of 4 is 16 4 2 16 and the square root of 16 16. Show activity on this post. Square Root 1 To 100 Value Of Square Roots From 1 To 100 Pdf The smallest value in the domain is zero.

So lets think about what this is saying. Sqrt x2 sqrt 4 x2. There is no real number whose square is.

We say this because the root of a negative number cant be any real number. The square root is an inverse operation of squaring a number. If n is a number then its square is represented by n raised to the.

To indicate that we want both the positive and the negative square root of a radicand we put the symbol read as plus minus in front of the root. Answer 1 of 5. The other explanation for why there is a on the one side of the equation is very much more technical in a mathematical definition-based way.

If we have a positive number b then its square roots are written as shown in Figure 1. The answer will show you the complex or imaginary solutions for square roots of negative real numbers. Let us say m is a positive integer such that mm m 2 m.

Definition of Square Root. Given a positive real number a there are two solutions to the equation x2 a one is positive and the other is negative. 9 3.

9 3. The positive number when it is to be multiplied by itself represents the square of the number. If youre familiar with negative numbers you know that theres also a negative square root but when you just see this symbol that means the positive square root.

The negative square squared will give the same positive result as the principal square root. The negative square root of b. A square root is written with a radical symbol and the number or expression inside the radical symbol below denoted a is called the radicand.

When we take the square root of either side we get the following. The radical sign means we are taking the positive square root of given equation. Hence the nature of the roots α and β of equation ax 2 bx c 0 depends on the quantity or expression b 2 4ac under the square root sign.

The behavior of the square root function when extended to the domain of all real numbers positive reals negative reals and 0 precisely mirrors the argument made above. A positive number has two square roots. Zero has one square root which is 0.

Sidharth KillerBunny. The square root of 100. For example 5 and 5 are both square roots of 25 because.

Technically there is not a square root for negative numbers so you can multiply the square number. Squaring a positive number gets a positive result. Square of zero is zero.

To indicate that we want both the positive and the negative square root of a radicand we put the symbol read as plus minus in front of the root. Given a positive real number a there are two solutions to the equation x 2 a one is positive and the other is negative. Zero has one square root which is 0.

5 x 5 25 and -5 x -5 25. The variable x could equal well have either one of those values. As a side issue this goes some way to explaining why you cannot have the square root of a negative number there is more about this in our page on Special Numbers and Concepts.

The square root of any number is equal to a number which when squared gives the original number. For example the square of 2 is 4 and the square root of 4 is 2. If we simply say taking square roots on both sidesthen we apply a before radical signas I said sign means positive square rootso in order to get negative one also we apply that sign.

Say x 2 -1 is a quadratic equation. Squaring a negative number also gets a positive result. Here this symbol we used to represent the root of numbers is termed as radical.

Because a negative times a negative gives a. The square root of a negative number is a complex number. Whereas square root of a number is value which on getting multiplied by itself gives the original value.

One is positive and one is negative. Sure enough the very first thing we encounter in the prompt is a variable squared and when we solve for x we have to account for both roots. But even with its domain extended x sqrtx x is similarly defined so that it is still a function.

For example 64 8 8 so 64 is undefined. In this video I find the square root of negative numbers. The square root of 49 is positive 7 and negative 7 we write this as 7.

When we square a number then take the square root we may not end up with the number we started with. So the negative square root is the same number as the principal square root but the negative version. This is asking us find the number the positive.

Inputs for the radicand x can be positive or negative real numbers. Use this calculator to find the principal square root and roots of real numbers. Hence both are vice-versa methods.

The square root of the square of any positive number gives the original number. Suppose we are given the equation x2 4 and we are told to solve. The reason that we cant have the square or quartic root of a negative number is that two negatives always cancel to give a plus.

Here a b and c are real and rational. The negative solution of x2 a is a we know that if x satisfies x2 a then x2 x2 a. In other words the square root is an operation that undoes an exponent of 2.

Negative number negative number positive number. Squares are the numbers generated after multiplying a value by itself. A square root is written with a radical symbol and the number or expression inside the radical symbol below denoted a is called the radicand.

We can write 125 as the product of 3 negative 5s. In mathematics a square root function is defined as a one-to-one function that takes a positive number as an input and returns the square root of the given input number. So the square root of a negative number is undefined.

A square root of a number x is such that a number y is the square of x simplify written as y 2 x. Up to 8 cash back For any square roots we take as part of our solution we are liable to account for both the positive and negative roots. X 2 4.

The principal square root is the nonnegative number that when multiplied by itself equals latexalatex. 5 5 25. The negative solution of x 2 a is a we know that if x satisfies x 2 a then x 2 x 2 a therefore because a is a solution so is.

The principal square root is the positive version. Positive number negative number negative number. We denote the positive root which we often call the square root by a.

Hence 3125 5. The square root is the number that is. When you see it like this this means the positive square root.

We denote the positive root which we often call the square root by a. The answer will also tell you if you entered a perfect square. The answer to a square root mathematically is positive or negative.

The square root could be positive or negative because multiplying two negative numbers gives a positive number. This is because when negative values are squared the product is a positive as well since a negative times a. The square root obtained using a calculator is the principal square root.

This is because 7 7 49 and -7 -7 49. In fact we end up with the absolute value of the number. X 2 x That also happens for all even but not odd.

Square Roots

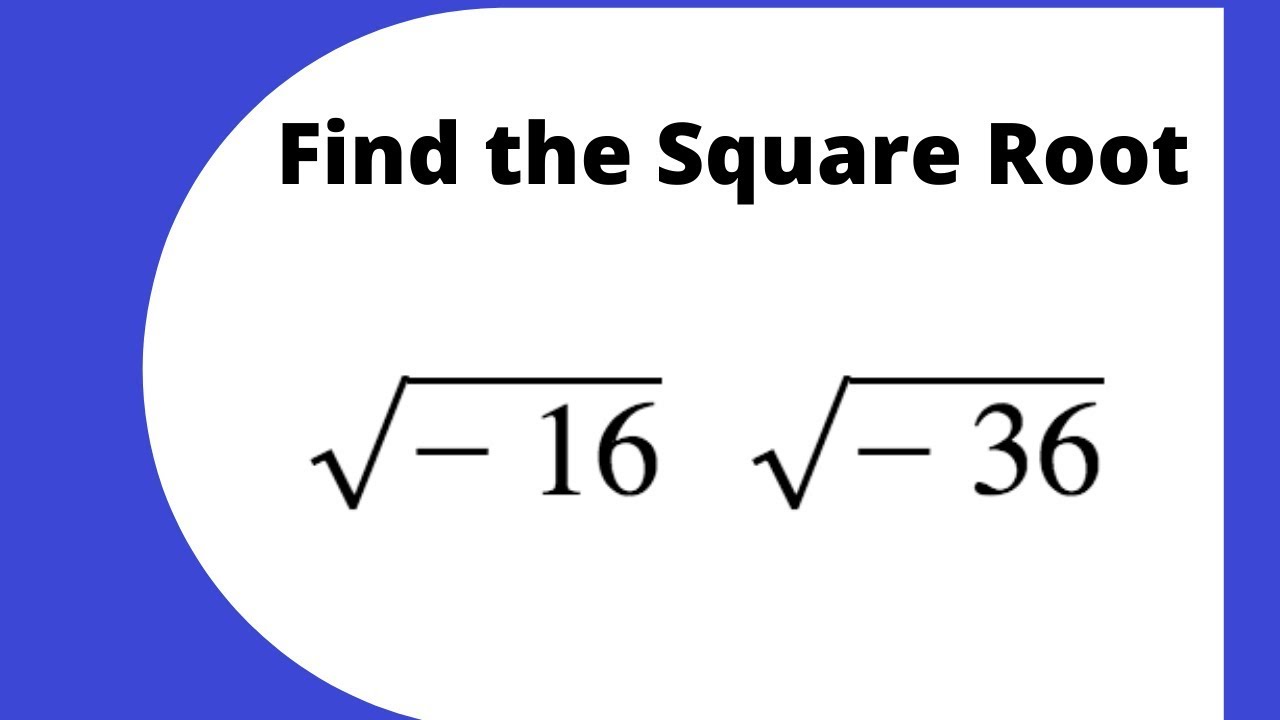
Find The Square Root Of Negative Numbers Youtube